Book Your Free Demo Now!
What are algebra formulas and how to use these?
Algebra is considered as a division of Maths which compacts with relations, operations and their constructions. It is considered as the best building wedges of maths and is used in a variety of applications in our routine life. In addition to importance as a main subject in our schools, Algebra used in doing Mathematical calculations also assists students in getting detailed understanding of other advanced branches of mathematics like Arithmetic, Calculus, Geometry, among others.
Through this write-up, we want students to understand more about Algebra, concepts and algebra formulas linked to it in an interesting and fun learning way. Students can easily learn algebra basic formulas to do the algebraic questions in case they are well-versed with Algebra and formulas used in everyday time for solving equations.
Concepts linked with Algebra
In the elementary Algebra, there are many instructions and operations on numbers like:
a) Add
b) Subtraction
c) Product
c) Product
e) Equation solving techniques
f) Variables
g) Functions
h) Polynomials
i) Algebraic Expressions
There is no limit on the difficulty of all these ideas as far as its all about Algebra. Newer thoughts and concepts may further be added to boost the education level. All these concepts are very valuable and reasonable if taught properly.
Some important algebra formulas with solutions:
1. a2– b2= (a+b)(a-b)
a=3, b= 2
3 (2)– 2(2)= (3+2)(3-2)
9-4=5
5=5
2. (a+b)2= a2 + 2ab+b2
a= 2, b= 1
(2+1) 2= 22 + 2(2)(1)+ (1)2
(3) 2= 4+4+1
9=9
3. a2+b2= (a+b)2 – 2ab
a= 2, b= 3
(2) 2 +(3)2 = (2+3)2 – 2(2)(3)
4+9= (5)2 -12
13= 25-12
13=13
Here is a list of Algebraic formulas
1. a2 – b2 = (a – b)(a + b)
2. (a + b)2 = a2 + 2ab + b2
3. a2 + b2 = (a + b)2 – 2ab
4. (a – b)2 = a2 – 2ab + b2
5. (a+b+c)2= a2 + b2+ c2+ 2ab + 2bc + 2ac
6. (a + b)3 = a3 + 3a2 b + 3ab2 + b3 = (a + b)3 = a3 + b3 + 3ab(a + b)
7. a3 – b3 = (a – b)(a2 + ab + b2)
8. a3 + b3 = (a + b)(a2 – ab + b2)
9. a4 – b4 = (a – b)(a + b)(a2 + b2)
You can take Ziyyara online classes to understand these formulas.
4. (a-b)2= (a)2 – 2ab + (b)2
a= 5, b= 3
(5-3) 2= (5)2– 2(5)(3) + (3)2
(2) 2= 25-30=9
4= 34-30
4=4
5. (a+b+c)2= a2 + b2+ c2+ 2ab + 2bc + 2ca
a=2, b= 1, c=3
(2+1+3) 2= (2)2 + (1)2 + (3)2 + 2(2)(1)+ 2(1)(3) + 2(2)(3)
(6) 2= 4+1+9+4+6+12
36= 36
6. (a+b)3= a3 + 3a2 b + 3ab2 + b3
a= 2, b= 3
(2+3) 3= (2)3 +3(2)2 (3) + 3(2)(3)2 + (3)3
(5) 3= 8 +3(4)(3)+ 3(2)(9) + 27
125= 8+36+54+27
125= 125
7. A3– b3= (A-b) (a2+ ab+b2)
a= 5, a=2
(5) 3-(2)3= (5-2) ( (5)2 + (5)(2) + (2)2 )
125-8= (3) (25+10+4)
117= 3(39)
117=117
8. A3 +b3 = (a+b) (a2– ab+b2)
a=1, b=2
(1) 3 + (2)3 = (1+2) ( (1)2 – (1)(2) + (2)2 )
1+8= 3( 1-2+4)
9= 3(3)
9=9
9. A4– b4= (a+b)(a-b)(a2+b2)
a=3; b=2
(3) 4 – 2(4) = (3-2) (3+2) ( (3)2 + (2)2 )
81-16= (1)(5)(9+4)
65= 5(13)
65=65
Algebra basic formulas are used in day to day life, from using them in getting the volumes of shapes to converting their units while measuring, we all use different formulas to get the results. Knowing how to use math formulas and rearranging them is a must which you one can learn by getting assistance from qualified teachers at Ziyyara who offer one to one online classes to the learners.
There are a few algebra formulas class 10 that are used frequently at the time of doing algebraic manipulations and working through mathematical applications. There are different ways of using these algebra formulas even while doing something different than algebra. But before applying these formulas somewhere else, it is important to understand the letters and symbols used in the formulas which is taught by the online teachers at Ziyyara.
Unlock Expert Guidance – Book Help Now!
Avail now by providing the below details

Some of the math algebra formulas with explanations and Algebra Basics are mentioned below:
While learning Algebra maths formulas, it is imperative to have detailed understanding of the mathematical terms that are used. Let’s quickly revise these Algebraic terms before doing the questions as it will make you familiar with some of the important and widely used Algebraic terms.
a) Equation
An equation is explained as a statement which includes symbols, numbers, and mathematical operators (Addition, Subtraction, Multiplication, Division etc) that represent the equality of two maths expressions. The fairness of the two terms is mentioned by a symbol “=” which is pronounced as “is equal to”. For instance: 4X + 9 = 16 is a calculation.
b) Variable
A variable is a symbol which mentions a quantity in any algebraic equation. It is that value which can be amended with time and scope of the concerned problem. For instance, in a mathematical equation 3X + 7 = 17, here X is considered as the variable as it can change with time. Also in the case of an equation, X2 + 5XY – 6Y2, here both X and Y are considered as variables.
c) One Variable Equation
An equation where there is only one flexible is being referred to as One Variable Equation. Example 5X + 7 = 19 is an instance of one variable equation only.
d) Two Variable Equation
An equation connecting two variables is mentioned as a Two Variable Equation. For instance, 3X + Y = 15 is a Two Variable Equation where X and Y are two variables and in this equation, both X and Y have a power of 1. Hence this equation has a degree of 1 which is equal to the maximum power of the variable(s) involved. Another example is X2 + 5XY – 4Y2 = 27 is just a sample of a Two Variable Calculation of degree 2.
e) Polynomial Expression
polynomial expression is measured with the ones which involve more than one term with non-negative important exponents of a variable. Instances of polynomial expressions are ab+bc+ca, etc.
f) Exponent
Exponentiation is reflected as a maths equation written, where ‘a’ is measured as the base and n is referred as the power with positive number. So we can say that in the process of exponentiation, the number is continuously multiplied by the same number, and the exponent means the number of times it gets multiplied. For in X3, X is multiplied with the same number itself, to get the final result.
Why is it important to learn Algebra?
Learning all algebra formulas at the starting level is significant as it helps get a broader perspective of the same and will prove a resourceful branch of mathematics in gaining broader experience.
If school students spend a few hours only practicing Algebra regularly, they can easily get the real insights of algebra and can solve the algebraic problems easily and more effectively. Once the essentials are clear, schoolchildren can practice the same by solving advanced, algebraic, abstract, commutative, etc. equitation. By understanding algebra basic formulas, you not only will be able to understand an academic program or course effectively but can use your skills in the future as well.
Learning Algebra is not a rocket science and in case you study harder from the beginning, can easily grasp all the information. The reputation of formulas of algebra in real life is vibrant and thus it is important to choose the right platform which helps learn algebra formulas with examples in a more fun filled way. Ziyyara is one such platform which helps in making the mathematical concepts easy. It offers one-to-one online classes to the beginners so that they can learn more about formulas of algebra which is used not only by mathematicians but by academicians, educationists, scholars, etc.
Like? Share it with your friends
-
Facebook
-
Twitter
-
Linkedin
-
Whatsapp
-
Reddit
Recent Posts
Quran Tajweed Online Learning
Top 50 Questions of Plant and Animal
Top 50 Questions of Carbon and its
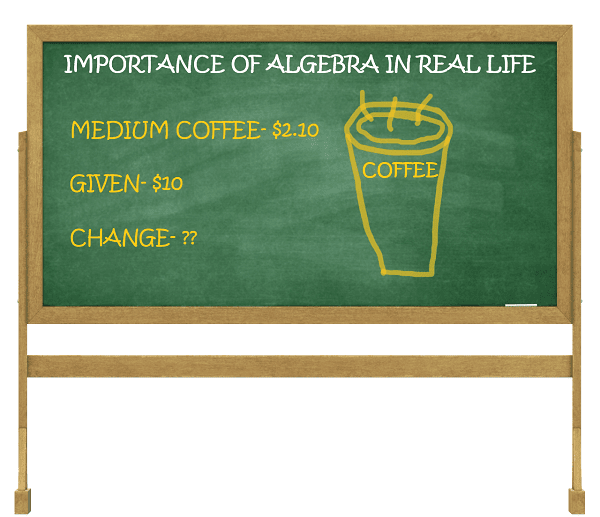
What is the Importance of Algebra in